8

My apologies in advance if this questions is better suited to be migrated elsewhere.

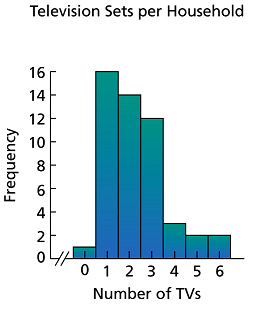
In a standard (X,Y) Cartesian line/dot plot the axis should be placed in a contextually logical place. In a graph presenting real world quantification with only real positive integers that are possible (>= 0) I am told that the only possible intersection for the axis is (0,0). Even it seems at the expense of clearly seeing a point at X=0...

I would like to ask if in academic publications there is any validity to permitting your reader to see all your points clearly by applying a subtle shift (padding the axis to the left). See figure included.

enter image description here

The opposition I am receiving to this concept stems from the inference that the y-axis being placed in a negative region gives rise to the possibility that negative values are possible, even though it's a physical impossibility in this context to go below 0 in terms of having a physical entity. But because the axis stops (without labels) at the y axis and doesn't carry past it (deeper (except for the tick mark at y=0) into the negative quadrant, it is obvious and better to see the point and it's error bars. The range is quite significant and so these error bars are hard enough to see.

Here are some of the consequences I see when I plot the axis at (0,0)

  • Point gets a little hard to see and the error bar almost entirely disapeers
  • Error bar caps look y axis ticks which make the y axis messy and the error bar almost invisible. Increasing thickness of error bars looks very messy.
  • Increasing point size could encompass the error bars in this particular context.
Improve this question
9
  • I'm sure some people will argue that this is better suited for Cross Validated, and others will disagree. To preempt the flags asking for migration, I would like to point out that given there may be some disagreement and as per this meta post, I'm not going to migrate this anywhere unless it's judged to be off-topic here (not just better somewhere else) and closed here first. (Unless, of course, the author prefers to migrate it.)
    – ff524
    Commented Dec 30, 2015 at 22:34
  • @ff524 Thanks for improving the title. I considered putting in on Cross Validated but I decided to put it here because I already know the letter of the rule. I think the best "advice oriented" publication guidance, and acceptable ways of interpreting these rules and applying them in consideration of the current context to achieve the best possible solution will be obtained here and will be experience based rather than rule based and supported as I might even advise if seeing the question on Cross Validated.
    – EngBIRD
    Commented Dec 30, 2015 at 23:07
  • 2
    Have you considered a Tufte range frame (like this)?
    – ff524
    Commented Dec 30, 2015 at 23:12
  • 1
    I personally would not have any problem with this representation. An alternative might be to have the y-axis on the righthand side instead.
    – Gerhard
    Commented Dec 31, 2015 at 0:05
  • 1
    With axes there's the implicit assumption that they intersect at zero. Anything else would be confusing. But if you have a frame surrounding the plot and the tick marks are put on the frame, this doesn't necessarily have to be so. There needs to be enough room for everything within the frame, then you put the tick marks as appropriate. Example. The two examples I linked use the default style of Mathematica. You'll see that in the framed example zero doesn't coincide example with the left frame.
    – Szabolcs
    Commented Dec 31, 2015 at 8:35

2 Answers 2

Reset to default
7

The point of a figure is to convey meaning and to do so in as easily understandable a way as possible. So, if you have a case where you think that the result is clearer if you offset the x-axis slightly, then do it. The guiding line should be: Does it help the reader understand better what you think the important point is than any other way of presenting the data?

Improve this answer
6

Is it valid to pad an axis slightly in order to enhance point visibility?

Yes. For example, in the textbook by Neil Weiss, Introductory Statistics, all of the examples including data in the 0-class are treated this way:

TV sets per household histogram

Note the symbol // on the horizontal axes in Figs. 2.3(a) and (b). This symbol indicates that the zero point on that axis is not in its usual position at the intersection of the horizontal and vertical axes. Whenever any such modification is made, whether on the horizontal or vertical axis, the symbol // or some similar symbol should be used to indicate that fact. (Weiss, 8E, Sec. 2.3)

While this is used throughout on the horizontal axis, what the reader is warned against is modifying the location of the zero on the vertical axis:

Figure 2.12(a) is an example of a truncated graph because the vertical axis, which should start at 0%, starts at 4% instead. Thus the part of the graph from 0% to 4% has been cut off, or truncated. This truncation causes the bars to be out of proportion and hence creates a misleading impression... Truncated graphs have long been a target of statisticians, and many statistics books warn against their use. (Weiss, 8E, Sec. 2.5)

Improve this answer
5
  • Presumably the issue you point out regarding the vertical axis only applies to bar graphs, though. The proportion of the OP's data points + error bars would not be affected by the origin of the vertical axis.
    – ff524
    Commented Dec 31, 2015 at 5:26
  • @ff524: That's debatable. I would plan to put 0 at the bottom of the y-axis by default (as the OP seems to expect) unless some very clear reason presented itself that I shouldn't. Frankly, the only case that comes to mind would be a non-proportional measure like temperature in Fahrenheit or Celsius.
    – Daniel R. Collins
    Commented Dec 31, 2015 at 5:43
  • 2
    Related: How to determine whether or not the y-axis of a graph should start at zero?
    – ff524
    Commented Dec 31, 2015 at 5:45
  • @ff524: I like that the top answer in that linked question indeed uses temperature as its example of when to use a nonzero vertical basis, which makes sense because it's (almost uniquely) a non-proportional measure.
    – Daniel R. Collins
    Commented Dec 31, 2015 at 6:59
  • 1
    Thanks a lot for your answer! I really liked it, but when I proposed it as an alternative it was rejected by my collaborators as "distracting", "busy", and "unconventional" (which I find a very poor excuse). Never-the-less, thanks for supporting it so well. I think this would be a good way to address the issue and keep everyone technically happy.
    – EngBIRD
    Commented Feb 17, 2016 at 2:00

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .