In mathematics, what distinguishes an exceptional undergraduate thesis for the rest? How do original contributions, accessibility of the topic (ie more or less prerequisites), novelty of the topic, etc factor in. Would it, for example, be better for an undergraduate to give an overview of well trotted areas or venture into less significant areas and try to pick some low haning fruit?
-
2Would it be better for what? For some students, it would be better preparation for graduate studies to learn important, established advanced topics in mathematics. For others, to get some research experience. Both are valuable in different ways.– KimballCommented Mar 10, 2015 at 15:32
1 Answer
For me: significant originality, even if that originality is expressed within the context set by the supervisor.
One cannot expect the student to understand what problems are interesting to the community, and which are feasibly solved by an undergraduate. But once the context is set by the supervisor, there can be significant room for originality within that context.
I was working on "locked polygonal chains" in 2D and 3D, and posed the question to my student of whether or not such chains could lock in 4D. The answer was provided in her undergraduate thesis, and subsequently published in a journal: No.
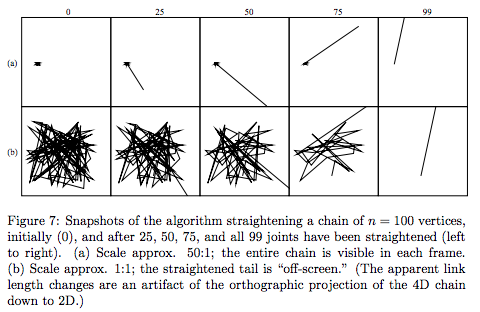
The proof was very much an equal-footing collaboration, but she was inventive and precise throughout. Especially on the algorithm illustrated above, she was ahead of me.
Roxana Cocan and J. O'Rourke. "Polygonal Chains Cannot Lock in 4D." Comput. Geom. Theory Appl., 20 (2001) 105-129.
-
If you research & write it as if it was a Master's thesis, it should be a significantly better than average Bachelor's thesis. (Did that, actually. Was told that it would actually hae been good enough to cover me if I just wanted to go another year and get the MS. Sometimes regret not having done so, but in retrospect I was in the wrong half of the department anyway.)– keshlamCommented Mar 11, 2015 at 3:33
